
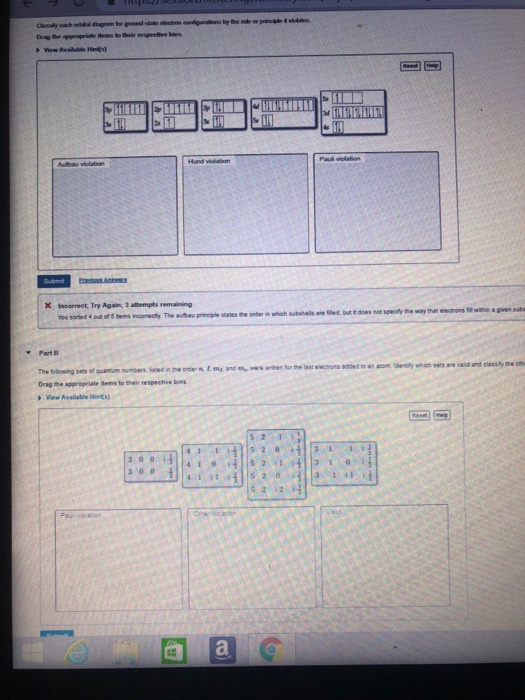
The h orbital, in which the azimuthal quantum number equals 5.The g orbital, in which the azimuthal quantum number equals 4.The f orbital, in which the azimuthal quantum number equals 3.

The d orbital, in which the azimuthal quantum number equals 2.The p orbital, in which the azimuthal quantum number equals 1.The s orbital, in which the azimuthal quantum number equals 0.The atomic orbitals' simple names and the corresponding value of the azimuthal quantum number are listed below. Relation of Quantum Numbers Defining Atomic OrbitalsĪn atomic orbital's name is usually a combination of the principal quantum number (n) and the azimuthal quantum number (l). These four quantum numbers are the principal quantum number, the azimuthal quantum number, the magnetic quantum number, and the electron spin quantum number. Thus, by determining the values of the four quantum numbers that describe an electron, one can gain insight into any electron residing in any atomic orbital in a given atom. The value of the spin quantum number, denoted by the symbol 'ms,' provides insight into the electron spin. atomic orbitals with two electrons, each electron has an equal and opposite spin to the other. In completely occupied atomic orbitals, i.e. The magnetic quantum number (abbreviated 'ml')įurthermore, each atomic orbital can only hold a maximum of two electrons.The azimuthal quantum number (abbreviated 'l') is also known as the orbital angular momentum quantum number.The primary quantum number (represented by the symbol 'n').It is important to note that the properties of each atomic orbital are determined by the following quantum numbers: The mathematical form of the atomic orbital predicts the presence of an electron in such a region. It should be noted that the term "atomic orbital" can also refer to the physical space or region around an atom's nucleus where the probability of a specific electron being present is greatest. These mathematical functions are often used in quantum mechanics and atomic theory to find out the probability of finding an electron (belonging to an atom) in a specific area around the atomic nucleus. But in the first picture, only the bonding mo is gerade while the anti-bonding mo is ungerade I'm not getting how by rotating along the axis perpendicular to the inter-nuclear axis, the sign of the antibonding mo changes making it ungerade.Atomic orbitals are mathematical functions that provide information about the wave nature of electrons (or pairs of electrons) that exist around atomic nuclei. If I rotate about the first orbital about an axis perpendicular to the inter-nuclear axis, the smaller lobe goes to the right only the sign always remains same just the position of the lobe has been changed. Mo due to to overlapping of s-p orbitals: Now, it can be detected easily which MO, formed due to the overlapping of s-s or p-p orbitals, is gerade or ungerade as shown in these pics:īut what about the molecular orbitals formed due to the overlapping of s-p orbitals or p-d orbitals? If the sign of the lobes remains the same, the orbital is gerade, and if the sign changes, the orbital is ungerade. An alternative method for determining the symmetry of the molecular orbital is to rotate the orbital about the line joining the two nuclei and then rotate the orbital about the line perpendicular to this. J.D.Lee writes in his book Concise Inorganic Chemistry:


 0 kommentar(er)
0 kommentar(er)
